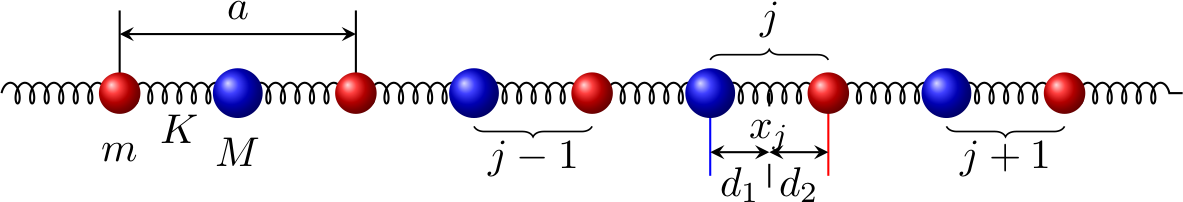
Plotly: 1D Diatomic Chain Dispersion

1D Diatomic Chain Dispersion

Consider a 1D chain with two atoms in the unit cell

1D diatomic chain. two sublattices. Generated by TikZ/LaTeX.

The coordinates of each atom in the cell

$\begin{equation} R^{j}_s(t) = x_j + d_s + u^{j}_s(t);\qquad s=1,2 \end{equation}$

where $x_j$ is the vector of the $j$ -th cell, $d_s$ is the relative vector of the $s$ -th atom in the cell, $u^j_s(t)$ is the displacement of the $s$ -th atom in the $j$ -th cell from its equlibrium position.

For the atoms in the $j$ -th cell, Newton’s law yields these euqations of motion

$\begin{align} m \frac{\mathrm{d}^2 u^{j}_1}{\mathrm{d}t^2} &= K (u^{j}_2 + u^{j-1}_2 - 2u^{j}_1) \\[6pt] M \frac{\mathrm{d}^2 u^{j}_2}{\mathrm{d}t^2} &= K (u^{j}_1 + u^{j+1}_1 - 2u^{j}_2) \end{align}$

where $m$ and $M$ are the masses of atom 1 and 2, respectively.

To find the solutions to the equations, we assume that all of the atoms move with the same frequency,

$\begin{align} u^{j}_1(t) &= \frac{A_q}{\sqrt{m}} e^{i(q{x_j} - \omega t)} \\[6pt] u^{j}_2(t) &= \frac{B_q}{\sqrt{M}} e^{i(q{x_j} - \omega t)} \end{align}$

Substitute this into the equations of motion, we have

$\begin{equation} \begin{pmatrix} \frac{2K}{m} - \omega^2 & \frac{-K}{\sqrt{mM}}(1+e^{-iqa}) \\ \phantom{.} \\ \frac{-K}{\sqrt{mM}}(1+e^{iqa}) & \frac{2K}{M} - \omega^2 \end{pmatrix} \begin{pmatrix} A_q \\ \phantom{a} \\ B_q \end{pmatrix} = 0 \end{equation}$

The equations will have a solution when the determinant of the matrix equals zero, i.e.

$\begin{equation} (\frac{2K}m - \omega^2) (\frac{2K}M - \omega^2) - 2\frac{K^2}{mM}(1 + \cos(qa)) = 0 \end{equation}$

This equation is quadratic and the solution can be easily found out

$\begin{equation} \omega_{\pm}^2 = {K\over Mm} \left[ (m + M) \pm \sqrt{m^2 + M^2 + 2mM\cos{(qa)}}\, \right] \end{equation}$

where the subscript $+$ / $-$ denote the optical/acoustic mode, respectively.

The maximum frequency is found ath $q=0$ of the optical mode

$\begin{equation} \omega_{\text{max}} = \sqrt{2K(m+M)\over mM} \end{equation}$

$\omega_{+}$

$\omega_{-}$ m/M = 0.500.000.100.200.300.400.500.600.700.800.901.00

Generated by Plotly

Plotly: 1D Diatomic Chain Dispersion

1D Diatomic Chain Dispersion

Further Reading

Angular Momentum in Solids

Plotly: Tight-binding Model for Graphene

Plotly: Spherical Harmonics

Trending Tags